2.2 多項(xiàng)式函數(shù)
看其他篇章到目錄選擇。
在Commons Math中的analysis.polynomials包中有所有的與多項(xiàng)式函數(shù)相關(guān)的類(lèi)和接口定義。這一篇主要從這個(gè)包分析，來(lái)研究一下多項(xiàng)式函數(shù)的應(yīng)用。
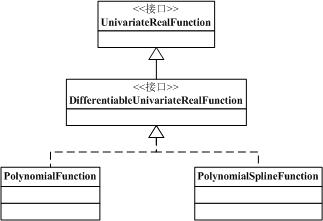
Polynomials包中沒(méi)有interface的定義，下屬含有5個(gè)類(lèi)：PolynomialFunction、PolynomialFunctionLagrangeForm、PolynomialFunctionNewtonForm、PolynomialSplineFunction和PolynomialsUtils。其中主要的只有PolynomialFunction和PolynomialSplineFunction，正如api doc中的介紹，PolynomialFunction類(lèi)是Immutable representation of a real polynomial function with real coefficients——實(shí)數(shù)多項(xiàng)式的表示；PolynomialSplineFunction類(lèi)是Represents a polynomial spline function.——樣條曲線(xiàn)多項(xiàng)式的表示。另外兩個(gè)表示拉格朗日和牛頓形式的多項(xiàng)式函數(shù)。而PolynomialsUtils類(lèi)中提供了幾個(gè)構(gòu)造個(gè)別（比如切比雪夫多項(xiàng)式）多項(xiàng)式的靜態(tài)方法。
我覺(jué)得最常用的應(yīng)該就是實(shí)數(shù)系數(shù)的多項(xiàng)式了，因此以PolynomialFunction類(lèi)為例來(lái)進(jìn)行分析。實(shí)數(shù)系數(shù)的多項(xiàng)式函數(shù)形如：f(x) = ax^2 + bx + c。PolynomialFunction類(lèi)實(shí)現(xiàn)了DifferentiableUnivariateRealFunction接口，因此必須實(shí)現(xiàn)value()和derivative()方法，并且實(shí)現(xiàn)該接口也表明這是一元可微分的實(shí)數(shù)函數(shù)形式。PolynomialFunction類(lèi)定義了一組final double coefficients[]作為多項(xiàng)式系數(shù)，其中coefficients[0]表示常數(shù)項(xiàng)的系數(shù)，coefficients[n]表示指數(shù)為n的x^n次項(xiàng)的系數(shù)。因此，這個(gè)類(lèi)所表達(dá)的多項(xiàng)式函數(shù)是這樣的：f(x)=coeff[0] + coeff[1]x + coeff[2]x^2 + … + coeff[n]x^n。它的構(gòu)造方法是PolynomialFunction(double [])就是接受這樣的coefficients數(shù)組作為系數(shù)輸入?yún)?shù)來(lái)構(gòu)造多項(xiàng)式的。這個(gè)是很好表達(dá)也很方便理解的。那么它的value(double x)方法是通過(guò)調(diào)用double evaluate(double[] coefficients, double argument)實(shí)現(xiàn)的，本質(zhì)用Horner's Method求解多項(xiàng)式的值，沒(méi)有什么技術(shù)難點(diǎn)，非常好理解的一個(gè)給定參數(shù)和函數(shù)求值過(guò)程。剩余定義的一些加減乘等操作，都是通過(guò)一個(gè)類(lèi)似public PolynomialFunction add(final PolynomialFunction p)這樣的結(jié)構(gòu)實(shí)現(xiàn)的。求導(dǎo)數(shù)的方法derivative()是通過(guò)這樣的一個(gè)微分操作實(shí)現(xiàn)的。見(jiàn)源碼：
1
protected static double[] differentiate(double[] coefficients) 
{
2
int n = coefficients.length;
3
if (n < 1) {
4 throw MathRuntimeException.createIllegalArgumentException("empty polynomials coefficients array");
5
}
6 if (n == 1) {
7 return new double[]{0};
8 }
9 double[] result = new double[n - 1];
10 for (int i = n - 1; i > 0; i--) {
11 result[i - 1] = i * coefficients[i];
12 }
13 return result;
14
}
15
測(cè)試代碼示例如下：
1/** *//**
2 *
3 */
4package algorithm.math;
5
6import org.apache.commons.math.ArgumentOutsideDomainException;
7import org.apache.commons.math.analysis.polynomials.PolynomialFunction;
8import org.apache.commons.math.analysis.polynomials.PolynomialSplineFunction;
9
10/** *//**
11 * @author Jia Yu
12 * @date 2010-11-21
13 */
14public class PolinomialsFunctionTest {
15
16 /** *//**
17 * @param args
18 */
19 public static void main(String[] args) {
20 // TODO Auto-generated method stub
21 polynomials();
22 System.out.println("-----------------------------------------------");
23 polynomialsSpline();
24 }
25
26 private static void polynomialsSpline() {
27 // TODO Auto-generated method stub
28 PolynomialFunction[] polynomials = {
29 new PolynomialFunction(new double[] { 0d, 1d, 1d }),
30 new PolynomialFunction(new double[] { 2d, 1d, 1d }),
31 new PolynomialFunction(new double[] { 4d, 1d, 1d }) };
32 double[] knots = { -1, 0, 1, 2 };
33 PolynomialSplineFunction spline = new PolynomialSplineFunction(knots,
34 polynomials);
35 //output directly
36 System.out.println("poly spline func is "+spline);
37 // get the value when x = 0.5
38 try {
39 System.out.println("f(0.5) = "+spline.value(0.5));
40 } catch (ArgumentOutsideDomainException e) {
41 // TODO Auto-generated catch block
42 e.printStackTrace();
43 }
44 // the number of spline segments
45 System.out.println("spline segments number is "+spline.getN());
46 // the polynomials functions
47 for(int i=0;i<spline.getN();i++){
48 System.out.println("spline:f"+i+"(x) = "+spline.getPolynomials()[i]);
49 }
50 //function derivative
51 System.out.println("spline func derivative is "+spline.derivative());
52 }
53
54 private static void polynomials() {
55 // TODO Auto-generated method stub
56 double[] f1_coeff = { 3.0, 6.0, -2.0, 1.0 };
57 double[] f2_coeff = { 1.0, 2.0, -1.0, -2.0 };
58 PolynomialFunction f1 = new PolynomialFunction(f1_coeff);
59 PolynomialFunction f2 = new PolynomialFunction(f2_coeff);
60 // output directly
61 System.out.println("f1(x) is : " + f1);
62 System.out.println("f2(x) is : " + f2);
63 // polynomial degree
64 System.out.println("f1(x)'s degree is " + f1.degree());
65 // get the value when x = 2
66 System.out.println("f1(2) = " + f1.value(2));
67 // function add
68 System.out.println("f1(x)+f2(x) = " + f1.add(f2));
69 // function substract
70 System.out.println("f1(x)-f2(x) = " + f1.subtract(f2));
71 // function multiply
72 System.out.println("f1(x)*f2(x) = " + f1.multiply(f2));
73 // function derivative
74 System.out.println("f1'(x) = " + f1.derivative());
75 System.out.println("f2''(x) = "
76 + ((PolynomialFunction) f2.derivative()).derivative());
77
78 }
79
80}
81
輸出如下：
f1(x) is : 3.0 + 6.0 x - 2.0 x^2 + x^3
f2(x) is : 1.0 + 2.0 x - x^2 - 2.0 x^3
f1(x)'s degree is 3
f1(2) = 15.0
f1(x)+f2(x) = 4.0 + 8.0 x - 3.0 x^2 - x^3
f1(x)-f2(x) = 2.0 + 4.0 x - x^2 + 3.0 x^3
f1(x)*f2(x) = 3.0 + 12.0 x + 7.0 x^2 - 15.0 x^3 - 8.0 x^4 + 3.0 x^5 - 2.0 x^6
f1'(x) = 6.0 - 4.0 x + 3.0 x^2
f2''(x) = -2.0 - 12.0 x
-----------------------------------------------
poly spline func is org.apache.commons.math.analysis.polynomials.PolynomialSplineFunction@69b332
f(0.5) = 2.75
spline segments number is 3
spline:f0(x) = x + x^2
spline:f1(x) = 2.0 + x + x^2
spline:f2(x) = 4.0 + x + x^2
spline func derivative is org.apache.commons.math.analysis.polynomials.PolynomialSplineFunction@173a10f
PolynomialFunction類(lèi)也是重寫(xiě)了toString方法和hashCode和equals方法的。
PolynomialSplineFunction類(lèi)是多項(xiàng)式樣條函數(shù)，樣條是一種特殊的函數(shù)，由多項(xiàng)式分段定義。表示了一個(gè)由多個(gè)多項(xiàng)式組成的樣條曲線(xiàn)。它的實(shí)現(xiàn)主要是內(nèi)部定義了一個(gè)多項(xiàng)式函數(shù)組PolynomialFunction polynomials[]和一個(gè)樣條分界節(jié)點(diǎn)數(shù)組double knots[]。這兩個(gè)內(nèi)部成員分別表示什么呢？分界節(jié)點(diǎn)表示整條曲線(xiàn)對(duì)應(yīng)在x等于knots[i]的時(shí)候開(kāi)始使用其他多項(xiàng)式樣條，其構(gòu)造方法public PolynomialSplineFunction(double knots[], PolynomialFunction polynomials[])完成這樣的功能。
舉例來(lái)說(shuō)，一個(gè)多項(xiàng)式樣條函數(shù)就是一個(gè)分段函數(shù)：
X^2+x [-1,0)
F(x) = x^2+x+2 [0,1)
X^2+x+4 [1,2)
當(dāng)然，構(gòu)造方法中的參數(shù)，knots[]數(shù)組必須是遞增的。
可以看到，直接輸出PolynomialSplineFunction是多么丑陋啊~~，因?yàn)樗鼪](méi)有重寫(xiě)toString方法。同樣，它的導(dǎo)數(shù)也是一樣的丑陋。其中如果給定的值不在定義域內(nèi)，value方法還拋出異常ArgumentOutsideDomainException。
最后PolynomialFunctionLagrangeForm和PolynomialFunctionNewtonForm類(lèi)完成的其實(shí)是多項(xiàng)式插值的功能，放到下一節(jié)研究的。
相關(guān)資料：
多項(xiàng)式：http://zh.wikipedia.org/zh-cn/%E5%A4%9A%E9%A1%B9%E5%BC%8F%E5%87%BD%E6%95%B0#.E5.A4.9A.E9.A0.85.E5.BC.8F.E5.87.BD.E6.95.B8.E5.8F.8A.E5.A4.9A.E9.A0.85.E5.BC.8F.E7.9A.84.E6.A0.B9
樣條函數(shù)：http://zh.wikipedia.org/zh-cn/%E6%A0%B7%E6%9D%A1%E5%87%BD%E6%95%B0
Horner Methods：http://mathworld.wolfram.com/HornersMethod.html
Commons math包：http://commons.apache.org/math/index.html