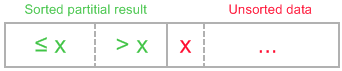
Insertion SortInsertion sort belongs to the O(n2) sorting algorithms. Unlike many sorting algorithms with quadratic complexity, it is actually applied in practice for sorting small arrays of data. For instance, it is used to improve quicksort routine. Some sources notice, that people use same algorithm ordering items, for example, hand of cards. AlgorithmInsertion sort algorithm somewhat resembles selection sort. Array is imaginary divided into two parts - sorted one andunsorted one. At the beginning, sorted part contains first element of the array and unsorted one contains the rest. At every step, algorithm takes first element in the unsorted part and inserts it to the right place of the sorted one. Whenunsorted part becomes empty, algorithm stops. Sketchy, insertion sort algorithm step looks like this:
becomes
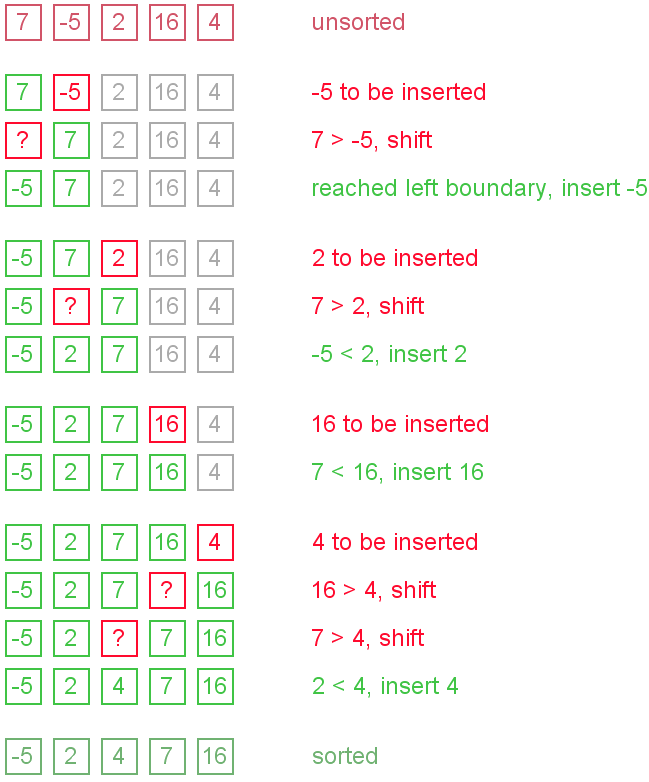
The idea of the sketch was originaly posted here. Let us see an example of insertion sort routine to make the idea of algorithm clearer. Example. Sort {7, -5, 2, 16, 4} using insertion sort.
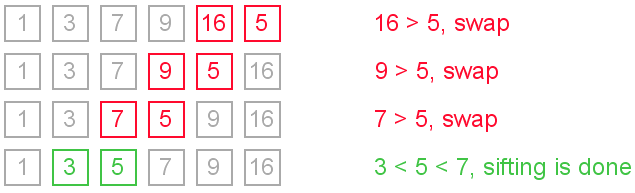
The ideas of insertionThe main operation of the algorithm is insertion. The task is to insert a value into the sorted part of the array. Let us see the variants of how we can do it. "Sifting down" using swaps The simplest way to insert next element into the sorted part is to sift it down, until it occupies correct position. Initially the element stays right after the sorted part. At each step algorithm compares the element with one before it and, if they stay in reversed order, swap them. Let us see an illustration.
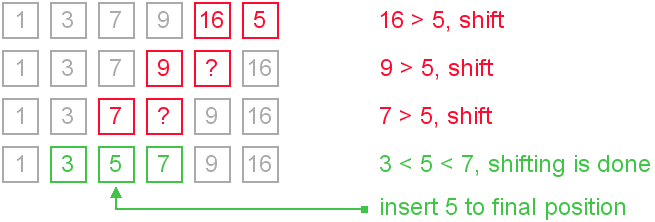
This approach writes sifted element to temporary position many times. Next implementation eliminates those unnecessary writes. Shifting instead of swappingWe can modify previous algorithm, so it will write sifted element only to the final correct position. Let us see an illustration.
It is the most commonly used modification of the insertion sort. Using binary searchIt is reasonable to use binary search algorithm to find a proper place for insertion. This variant of the insertion sort is calledbinary insertion sort. After position for insertion is found, algorithm shifts the part of the array and inserts the element. This version has lower number of comparisons, but overall average complexity remains O(n2). From a practical point of view this improvement is not very important, because insertion sort is used on quite small data sets. Complexity analysisInsertion sort's overall complexity is O(n2) on average, regardless of the method of insertion. On the almost sorted arrays insertion sort shows better performance, up to O(n) in case of applying insertion sort to a sorted array. Number of writes is O(n2) on average, but number of comparisons may vary depending on the insertion algorithm. It is O(n2) when shifting or swapping methods are used and O(n log n) for binary insertion sort. From the point of view of practical application, an average complexity of the insertion sort is not so important. As it was mentioned above, insertion sort is applied to quite small data sets (from 8 to 12 elements). Therefore, first of all, a "practical performance" should be considered. In practice insertion sort outperforms most of the quadratic sorting algorithms, like selection sort or bubble sort. Insertion sort properties
Code snippetsWe show the idea of insertion with shifts in Java implementation and the idea of insertion using python code snippet. Java implementationvoid insertionSort(int[] arr) { int i,j,newValue; for(i=1;i<arr.length;i++){ newValue = arr[i]; j=i; while(j>0&&arr[j-1]>newValue){ arr[j] = arr[j-1]; j--; } arr[j] = newValue; } Python implementationvoid insertionSort(L) { for i in range(l,len(L)): j = i newValue = L[i] while j > 0 and L[j - 1] >L[j]: L[j] = L[j - 1] j = j-1 } L[j] = newValue } } |
|
|||
|
|
|||
隨筆分類
隨筆檔案
搜索
積分與排名
- 積分 - 30958
- 排名 - 1362
最新評論

- 1.?re: Tomcat 6.0配置數(shù)據(jù)源(DBCP)和JNDI使用
- 嗯 萬分感謝 ,但問下在自己配置的數(shù)據(jù)源中 factory屬性必須添加嗎?我這里有一個apps就沒有添加factory屬性,沒有問題。
- --曹夢
- 2.?re: 二分查找的優(yōu)化和完備[未登錄]
- 評論內(nèi)容較長,點擊標題查看
- --ray
- 3.?re: 二分查找的優(yōu)化和完備
- 評論內(nèi)容較長,點擊標題查看
- --ytl
- 4.?re: 二分查找的優(yōu)化和完備
- 沒必要用遞歸吧,還可以優(yōu)化,展開成循環(huán)。
- --dennis
- 5.?re: 二分查找的優(yōu)化和完備
- mid比end小吧,end是int型,mid怎么會溢出呢
- --嚕嚕